Why did I get access to the wallet?

Question

I generated a 12-word BIP39 word sequence using a third-party library (not from the solana tools). Next, I went to the phantom mobile application and added a wallet by restoring it using a 12-word seed phrase.

Address: 8Y2XdtmZg6YRiYrzZxgpNw8yUg3JUJ7pUar5qbGjUiFf

My question is, why did I get access to the wallet? Does the wallet address initially exist on the blockchain and does the bip39 algorithm generate a unique passphrase to access this wallet address?

I apologize in advance for possibly very stupid questions and would appreciate any information.

Answer 1

The wallet algorithms work that way, that from a given seed phrase they always create the same set of addresses (accounts) where you store cryptocurrency or tokens. At the same time, the wallet will also create access keys to each individual address (the private keys) and protect them inside. It always creates the same set of addresses and private keys from one seed phrase. So if you will set up another wallet with the same seed phrase, it will create an identical copy of the first one. This is called a deterministic wallet, and almost all modern wallets work this way.

https://getcoinplate.com/blog/is-a-seed-phrase-the-same-as-a-private-key-the-ultimate-guide-to-private-keys-and-recovery-seed-phrases/

Answer 2

Short Answer: You have access to that particular "wallet" because only you have the corresponding Secret-Key for it. All wallets and Key-PAirs already exist, and have existed since the origins of the universe, when someone gets a key-pair, this person is not actually creating a wallet, but simply has possession of the random combination of seeds that when passed thru an algorithm, generate that pair.

Explanation:

The private key is a randomly generated scalar value, usually represented as an integer. The public key is a point on the curve, typically represented as a pair of coordinates (x, y). The public key is derived from the private key using mathematical operations defined by the elliptic curve equation.

So, ALL possible wallet addresses already exist, but no one knows their corresponding Secret-Keys ( derived from the secret seeds ) except the person or program that generated a Key-Pair that when ran thru a one-way hashing algorithm, produces the Public-Key. ( x,y coordinate in the plane, that is part of the Elliptic Curve being used )

Being points in a curve, numbers basically, they "already exist, always existed, since before human kind.. ".

There is a chance of more than one person having access to the same wallet if the seeds used are predictable or common. e.g. if 2 persons randomly used the same 12 words to generate the pair, both will get the same keys and thus, both will have access to the same wallet. This is why it is important to include a random element along with the chosen seeds. e.g. the 12 words + a big random number that will prevent that another person could choose the same 12 words.

In the pic below, you see your Public-Key, and it says it is "not inCurve". This is because without a corresponding Secret-Key, it is imposible to know if a coordinate is in the curve.

Note: I still don't know how the network can tell if an address is InCurve, after someone sends funds to it. ( e.g. https://solscan.io/account/8Y2XdtmZg6YRiYrzZxgpNw8yUg3JUJ7pUar5qbGjUiFf?cluster=devnet )

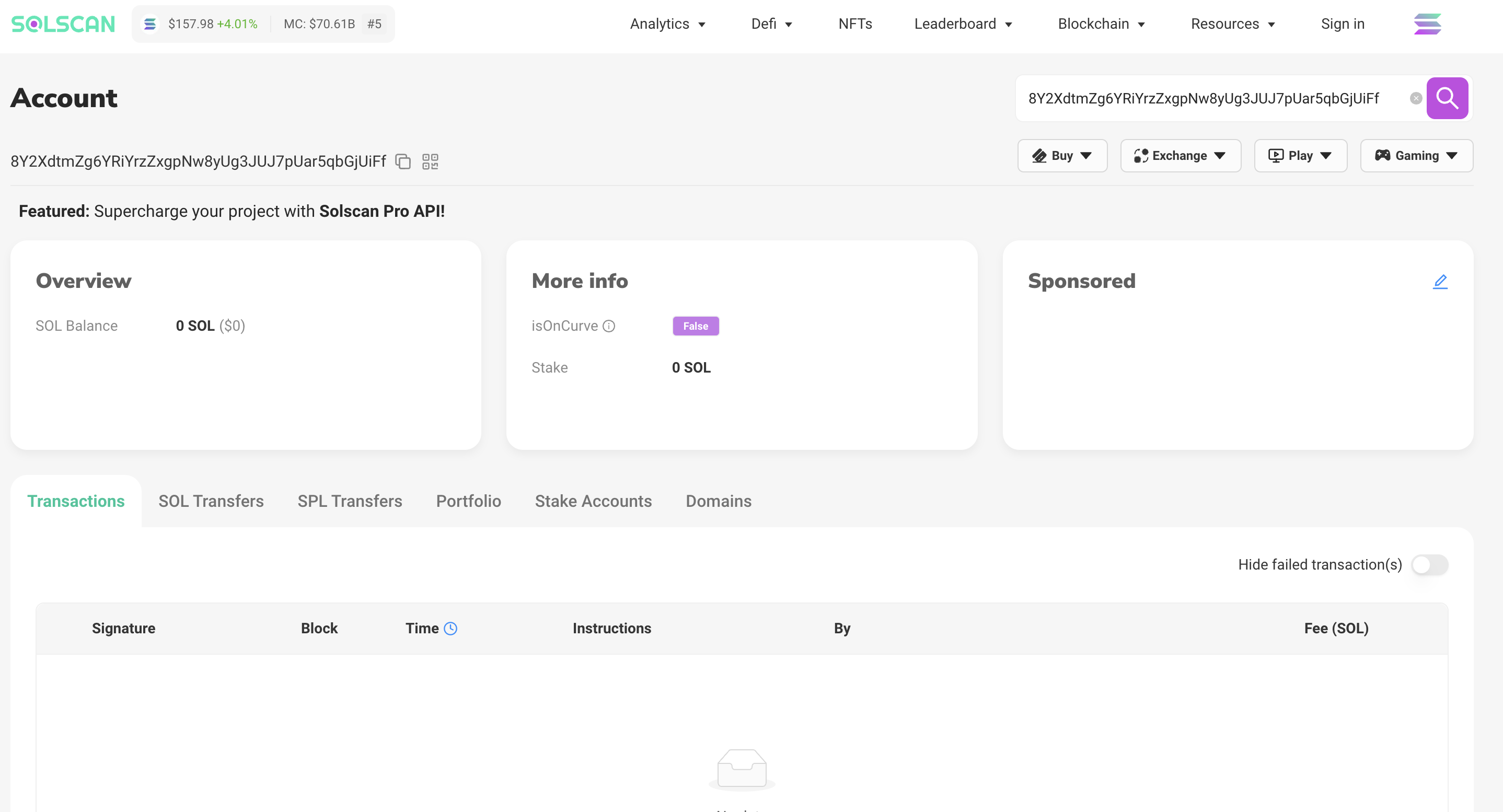
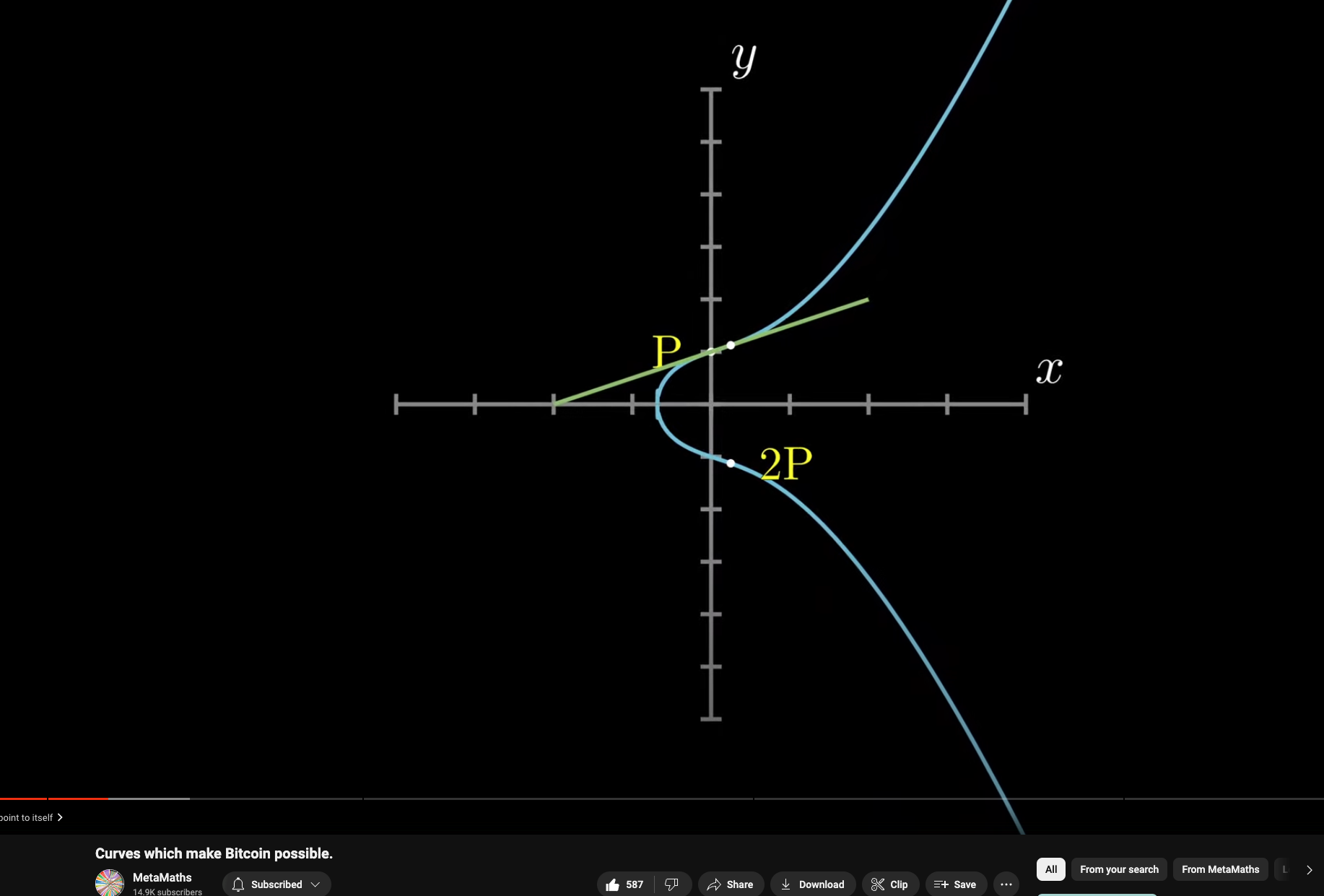
Here is a video that illustrates that very well: https://youtu.be/qCafMW4OG7s?si=HXiTWtcxm1eN110z